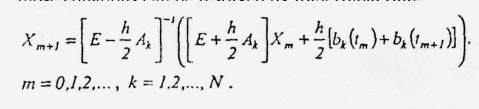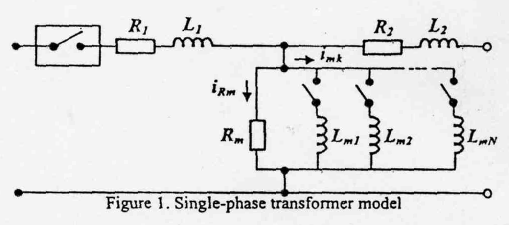
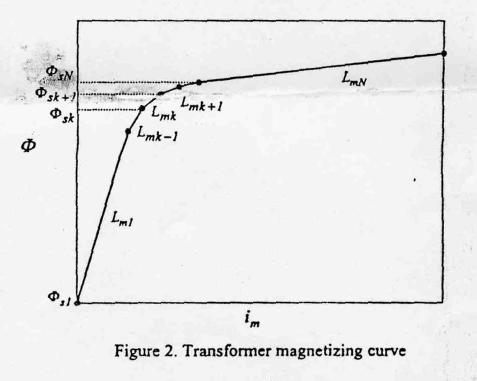

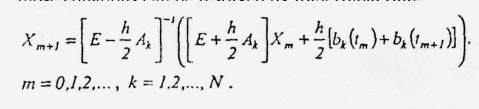
Abstract - In this paper is described a transformer model for low frequency electromagnetic studies, for frequencies from 0.1 Hz to 1 kHz. A non-linear magnetizing curve is presented with a finite number of linear regions. "Stiff" differential equations, which described behavior of transients, are solved by trapezoidal rule. The developed algorithm is successfully applied in low frequency transients, where the main subject of analysis is a nonlinear transformer iron core: inrush current and ferroresonance. Results of the "program" were compared to results in MATLAB/PSB.