DonNTU Master's portal

Abstract |
"Definition of risks at dynamic management of the capital of the trader or portfolio manager " |
Abstract
Research
Abstract
While considerable evidence has been produced concerning the efficacy of trading rules in futures markets, the results have generally not allowed for the reinvestment of profits as might be observed for real traders. Similarly, the determination of the appropriate capital allocation required per futures contract traded has been largely unstructured so making reported percentage returns questionable. This paper provides evidence of the profitability of a simple and publicly available trading rule in five futures markets but more importantly incorporates the ability to reinvest any profits via the ‘Optimal f’ technique described by Vince (1990). The results indicate that money management in speculative futures trading plays a more important role in trading rule profitability than previously considered by providing dramatic differences in profitability depending on how aggressively the trader capitalises each futures contract.
Research
The history of gaming mathematics is indeed extensive wherein authors attempt to grapple with maximising returns from payoffs governed by probabilistic properties. Examples include Bernoulli’s work in the 18th century (in Sommer 1954), Latane (1959), Thorp and Walden (1966), Thorp (1966, 1969, 1974 and 1980) and Connolly (1999).
If we view the returns from futures trading as being a probabilistic process we can identify various characteristics about trading rule performance. These characteristics include percentage of winning trades, maximum loss, maximum profit, average size of wins/losses and so on. While these characteristics are readily observable in most games of chance, historical results can be modelled in futures trading from testing a trading rule over a given data set. Armed with this information we can apply concepts developed in gaming mathematics to the position size problem in futures trading.
At the optimal f, we find the rate of reinvestment that would maximise the geometric rate of return on the portfolio and so dominate all other betting strategies applied to trading activities. In one empirical study of the value of fixed fractional betting, Ziemba (1987) found that a fixed fraction approach dominated all other betting strategies when applied to a horse-racing data set. In the method proposed by Vince (1990) maximising the geometric rate of return is achieved by modelling the largest observed loss and trading the portfolio reinvestment rate on this basis and determining which multiple of that largest loss would have produced the largest return on the funds invested.
To maximise the geometric growth we need to identify the account capitalisation required for each futures contract that produces the highest Terminal Wealth Relative (TWR) to the original investment per futures contract for a range of f values. As the account capitalisation per contract is a function of the f value selected and the largest loss, it is defined as:
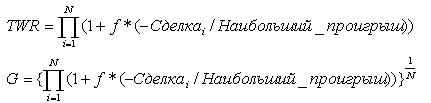 (1)
(1)
We take the negative value of the loss, hence the minus. Actually, this method implies that in the future the trade results will be about the same, but possibly in another order. Solving the TWR maximization, we find the f = fopt value, where the TWR function reaches its maximum. From fopt we define position size:
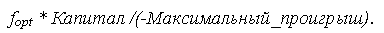 (2)
(2)
This method of estimating the optimal % of risk has been improved by Raplh Vince. While Kelly’s formula use only average values from past trades, Raplh Vince proposed to take into account all trades, solving the task of optimization of the relative end capital TWR as a function of f.
Besides, the distribution of trade results has a most profound influence on the fopt value. So fopt values for two strategies that in the end bring the same profit and have the same maximal loss may be very different.
The weak spot of the optimal f method is that it is fully based on the system’s historical results, on maximal loss to be exact. The risk level set when using fopt, means we‘ll never have a larger loss. Unlike gambling, where the outcomes are known and probabilities constant, in trading we have a multitude of random outcomes with undetermined probability of winning. The maximal loss is a nondescreasing step function, with random amplitude leaps occurring at random moments.
So, there is no real evidence to suppose that the maximal loss and maximal drawdown achieved will persist in the future. To calculate fopt it is possible to use in the TWR formula, instead of the maximal loss a value:
Max_Loss_Evaluation = Avg_Loss – 3.5 Standart_Deviation_of_Loss
But this doesn’t solve the problem yet. The outcome of a future trade is evidently random, so then the optimal f for is must also be random. The fopt value calculated from previous trades won't be really optimal for future trades, unless we turn to really reckless trading. Let's show an example of this.. So the so-called optimal f is really far from optimal.
Main lack of a method optimum f is that, it is completely based on historical results of system, namely, on the maximal loss. The risk level accepted at use fopt, means, that we never shall receive a heavy loss. Easier speaking, there are no bases to assume, that achieved the maximal loss and the maximal profitableness will stay those in the future.
 (2)
(2)
Figure 1 – Range of applicability for fîïò. Animation: 11 staff, a delay 70ìñ
This paper identified the importance of money management via the Optimal f technique for speculative traders in futures markets, when applying the turtle trading system. Some of the key findings highlighted the importance of capitalisation and reinvestment for returns to futures traders. While in some markets, such as US T-Bonds, the results showed that returns of greater than 100% were achievable, the results were far more dramatic in Corn futures where returns of around 2,000% were observed. Trade capitalisation played a far less important role in those markets where losses were produced and no amount of money management was able to convert the unprofitable trading rules into a winning position.
For empirical researchers, the conclusion that capitalisation and money management may play a role in determining the success or failure of speculative traders cannot be ignored. The results show that the propensity for account balances to be reduced to zero by undercapitalising futures trading positions is quite high and may contribute to the failure of many speculators. Finally, many works on the performance of technical trading models have reported results for a single futures contract for simplicity/comparability or for other reasons. The research presented here allows greater reconciliation between modelled results and the trading performance more likely to be encountered by traders with respect to risk attitudes observed in behavioural finance characteristics and the reinvestment of profits in speculative trading.
One limitation in this work is that the results were all obtained ex-post and so our conclusions
must contain an important caveat. Any profits generated in future tests may not reflect the past
trading performance and so the capitalisation issue may need to be treated more cautiously.
Several alternatives are apparent including the use of very strict stop-losses on trading positions
to ensure that the maximum observed loss is reflective of future trading activities. Similarly, the
use of f values of less than one implies that some account drawdown is experienced and must be
allowed for in the account capitalisation issue and so perhaps more conservative f values should
be applied.
Up