DEVELOPMENT OF THE METHOD PREVENTION OF INTERNAL CRACKS IN PROCESS OF SOFT REDUCTION CONTIUOUSLY CAST BLOOMS

Yevgeny Smirnov ^a, Vitaly Sklyar ^b

^aDepartment of Pressure Metals Processing, Donetsk national technical university, 58, Artyoma Street,83000 Donetsk, Ukraine omd@fizmet.donntu.ru,

^bDepartment of Pressure Metals Processing, Donetsk national technical university, 58, Artyoma Street,83000 Donetsk, Ukraine, fan-f1@yandex.ru

In article the description of a new two-stage method of deformation continuously cast blooms at a stage of incomplete crystallization (patent UA №75537) is given, and also results of its complex research by methods of mathematical and physical modeling with reference to conditions of manufacture continuously cast blooms by section 335х400 mm are submitted. Results of computing experiment with use of the developed mathematical model realized with use of the finite - element method, have allowed to specify the mechanism of influence of chemical compound casting steel, parameters of process casting and external deformation influence on resulting physical-geometrical condition continuously cast blooms, and formed in metal stress - deformed condition. Rational parameters of deformations process which providing a minimum level of occurrence disruption of continuity is determined. Physical modeling of process with use of a new method (patent UA №77236) has allowed to identify results of numerical modeling, and also to develop methodology of research new technology on the basis of principles mechanics of deformation a solid body. By complex researches is shown, that in case realization of a new method achievable efficiency of penetration of deformation in the field of the metal, adjoining with front of crystallization, on 15 … 35 % are higher, than in case of deformation blooms by the smooth roll at the classical one-stage scheme of deformation.

1. INTRODUCTION

Steady increase of requirements to quality an axial zone continuously cast blooms stimulated in last time development of principles of its deformation at the end of solidifications with the purpose of suppression of axial porosity and segregation: soft reduction or mechanical soft reduction. The technology of soft reduction is one of the most effective ways of improvement quality of internal layers continuously cast blooms. In this case the central part of section of continuously cast blooms is in liquid or liquid-solid condition (mushy zone). At the same time, in practice now there are, at least, some original technical decisions for realization of this method.

One of ways to increasing of deformation of internal layers of continuously casting bloom is reduction of bloom on the stage of incomplete crystallization on width less than width of bloom. So, on one of such technologies, for reduction on the stage of incomplete crystallization a blooms section 400x250 мм was used the roll with width 250-300 мм, that allowed to weaken liquation and eliminate appearance of internal cracks [1].

Also by the increase of penetration of deformation is giving the surface of bloom certain form directly in the process of reduction. In accordance with this technology two opposite surface of bloom are poured off protuberant [2]. Further in the stage of reduction these surface will be reduced to the size of bulge.

It is necessary one of new methods to consider and developed, at the level of patent on an invention, at the Department of Pressure Metals Processing “Donetsk national technical university” process of the two-stage deformation of continuously cast blooms on the stage of incomplete crystallization. It is suggested to divide the deformation affecting on two stages, namely: on the first stage deform the central areas of continuously casting bloom on a width which is 80-90% from the width of liquid-solid phase, and on the second stage deform the before undeformed regional areas.

2. OBJECT AND MODELING CONDITIONS

With the use of method of finite-element modeling, a numeral experiment was executed and the comparative analysis of the two-stage and classic (flat roll) method of deformation of continuously cast blooms is made on the stage of incomplete crystallization, and also the possible size of the valid for one occasion reduction e_i is certain on the basis of analysis of the formed tensely-deformed state. A geometrical model was a half by a continuous cast bloom with 335x400mm section is cast in the bloom CCM at OJSC “Dneprovskiy Iron-and-Steel Integrated Works named after F. Dzerzhinsky”.

A calculation was carried out for three sections along metallurgical length of bloom in places, which correspond beginning, middle and end of area of reduction on the stage of incomplete crystallization, as shown in work [3]. During setting of block from 5 reduction mills, points will correspond the places of setting of mills 1, 3 and 5. Probed three steel grade: 09Г2С, 40X and steel 70.

As a criterion allowing to estimate the degree of penetration of deformation in the internal layers of the crystallized bloom the resulted degree of relative deformation of e_пр, which was determined on a next formula

(1)

where Dh_ф - the absolute height deformation of diphasic area;

Н_бл - the initial height of bloom.

3. DISCUSSION OF THE MODELING RESULTS

The got results, presented on a fig. 1, allowed to draw a conclusion that the size e_пр grows with increase of degree of reduction and diminishes as far as diminishing of maintenance of liquid-hard phase, in connection with growth of thickness the hardening constituent of bloom. Thus, on the second stage e_пр less than on 3-23 % what on the first. It is explained, that on the second stage considerable part of the attached reduction is expended on deformation of lateral walls of continuously casting bloom.

Comparing of findings to analogical, but at reduction in the smooth rolls allow to draw a conclusion that e_пр during realization of the two-stage deformation of continuously cast blooms on the stage of incomplete crystallization anymore on 50-56%, that testifies to greater efficiency of new method.

The results of research of the tensely-deformed state of continuously cast bloomов on the stage of incomplete crystallization (see of fig. 2) allowed to specify the size of maximum relative deformation e_i.

а) b)

Fig. 1. Dependence of the resulted deformation on the relative reduction and place of deformation on the first (а) and second (b) stages

Thus, to the maximum size то set its value at which intensity of tensions in this point excels the size of tensile strength at metal in it. Researches showed that limiting is a size of intensity of tensions in a solid constituent, joining to front of crystallization e_i. Thus, it stipulates the maximally possible size e_i at the level of 0,5%. It should be noted that in points, lyings on a surface bloom size of intensity of tensions anymore on 2 stages, that is explained greater deformation of lateral walls of bloom.

а) b)

Fig. 2. Dependence of size of intensity of tensions in the limiting point of transversal section of continuously casting bloom from steel of 09Г2С from the relative reduction and place of deformation on the first (a) and second (b) stages

4. THE PHYSICAL MODELING CONDITIONS

To the physical modeling the following independent values were used as experimental factors: Dh/H₀ – ratio of the reduction in thickness to the initial height of the sample, F₀/F – ratio of the port area simulating liquid-solid phase to the cross section and q=(Т_melt-Т)/Т_melt – ratio of the melting temperature T_melt and temperature of the external surface of the sample T to the melting temperature.

Plan of the experiment is given in Table 1.

Table 1

Levels and variability intervals of the parameters

Parameters	Variability interval	Basic level (0)	Upper level (+1)	Lower level (-1)
Dh/H0	0,025	0,055	0,080	0,030
F0/F	0,016	0,098	0,114	0,082
q=(Тпл-Т)/Тпл	0,046	0,300	0,346	0,254

Hollow plasticine samples at a scale 1:10 were made for the purpose of performing the field research. Size of the hole modeling the liquid phase corresponded to the metallurgical length of the ingot equaling to 13, 15 and 17m. Coordinate grid in the form of the circumferences which had to be used for the analysis of the deformation process was applied to the side and contact surfaces of the sample. The terms of experiment corresponded described in work [4] with the purpose of comparison results.

5. DISCUSSION OF THE EXPERIMENTAL RESULTS.

The type of the deformed physical model is resulted on a fig. 3. On the contact surface of physical model risks which left the sharp edges of the first reduction pair of rollers are present. It goes to show that the accepted form of edge of roller falls short of necessary and needs further adjustment.

Fig. 3. Original appearance of physical model after deformation

The estimation of efficiency of new chart of deformation by the offered criterion of efficiency is executed:

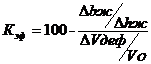(2)

where Dh_ж и Db_ж - accordingly absolute reduction and spreading of making physical model, imitating a liquid phase;

DV_деф - size of the displaced volume of bloom for two stages of deformation;

V₀ - volume of bloom of single length

The most efficiency is characterize the process of deformation on the area of metallurgical length of bloom, within the limits of which correlation of areas the liquid-sold and solod constituent of А_F close by 0,1(Fig 4) . Thus the size of coefficient of efficiency arrives at 90-96%.

Fig 4. Dependence of K_ef on the size of temperature gradient q in a solid constituent and А_F at е_I = 0,03

The estimation of forming of diphasic area by the coefficient of Db_ж/Dh_ж allowed to expose the mechanism of penetration of deformation in the middle of continuously casting bloom in the process of his two-stage reduction on the stage of incomplete crystallization. It is shown that on the first stage of deformation there is mainly bending of central area of the wrung out verge of bloom without substantial spreading of mushy zone and deformation of angular areas. At the same time, there is deformation of regional areas of the wrung out verge of bloom on the second stage. Thus a central area is involved in the process of deformation, and as a result there is total growth of height deformation.

The got results also allowed to confirm authenticity of results of calculations, got by a mathematical model.

CONCLUSION

The results of the analysis of a tensile condition continuous casting bloom are submitted in the field of realization "soft" reduction, for conditions of the two-phase process, received during modeling on the finite-element model. For verification of the before got results on research of this method by a mathematical design, planned and conducted experiment with the use of physical models. As a result of experiment the most rational parameters of process, which provide maximal efficiency, are certain. Exposed mechanism of penetration of deformation in the middle of continuously casting bloom during realization of process of the diphasic wringing out.

LITERATURE REFERENCES

1. К. ЦУЦУМИ и др. Легкое обжатие непрерывнолитого слитка валками с разной длинной бочки. Новости черной металлургии за рубежом. -1996. - № 1. - С. 52.

2. C. BYRNE. Mechanical soft reduction of billet. IMA Conference Series. – Oxford. - 1999.

3. СМИРНОВ Е.Н. Технологическое проектирование участка деформирования непрерыволитых блюмов на стадии неполной кристаллизации. Донбас-2020: наука і техніка - виробництву: Матеріали IV науково-практичної конференції. – Донецьк, ДонНТУ Міністерства освіти і науки. - 2008.- 597 с.

SMIRNOV E. N. and oth. Modeling of the stress and strain distribution in the process of reduction of the continuous cast ingots / 15th International Metallurgical & Material Conference “METAL 2006”. - Hradec nad Moravicн. Czech Republic [CD-ROM]., 2006. – P. 45-49.