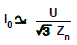Short circuit currents

SOURCE – Protection Guide By Merlin Gerin

---

Source of information: http://electrical-engineering-portal.com/short-circuit-currents

---

Introduction:

A short circuit is one of the major incidents affecting electrical systems. The consequences are often serious, if not dramatic:
• a short circuit disturbs the system environment around the fault point by causing a sudden drop in voltage.
• it requires a part of the system (often a large part) to be disconnected through the operation of the protection devices.
• all equipment and connections (cables, lines) subjected to a short circuit undergo strong mechanical stress (electrodynamic forces) which can cause breaks, and thermal stress which can melt conductors and destroy insulation.
• at the fault point, there is often a high power electrical arc, causing very heavy damage that can quickly spread all around.
Although short circuits are less and less likely to occur in modern well-designed, welloperating installations, the serious consequences they can cause are an incentive to implement all possible means to swiftly detect and attenuate them. The short circuit value at different points in the system is essential data in defining the cables, busbars and all breaking and protection devices as well as their settings.
Although short circuits are less and less likely to occur in modern well-designed, welloperating installations, the serious consequences they can cause are an incentive to implement all possible means to swiftly detect and attenuate them. The short circuit value at different points in the system is essential data in defining the cables, busbars and all breaking and protection devices as well as their settings.

1.Definitions:

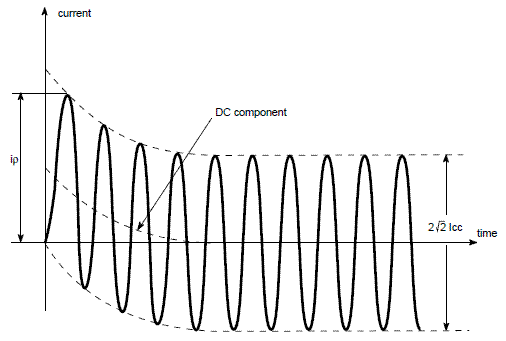
Short-circuit current at a given point in the system is expressed as the rms value Isc (in kA) of its AC component.
The maximum instantaneous value that short-circuit current can reach is the peak value Ip of the first half cycle. This peak value can be much higher than v2.Isc because of the damped DC component that can be superimposed on the AC component. This random DC component depends on the instantaneous value of voltage at the start of the shortcircuit and on the system characteristics.

2.Phase-to-phase shortcircuit

The Isc value of three-phase short circuit current at a point F within the system is:
In which U refers to the phase-to-phase voltage at point F before the fault occurs and Zcc is the equivalent upstream system impedance as seen from the fault point.
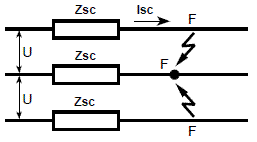
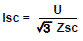
• in theory, this is a simple calculation; in practice, it is complicated due to the difficulty of calculating Zsc, an impedance equivalent to all the unitary impedances of series- and parallel-connnected units located upstream from the fault. These impedances are themselves the quadratic sum of reactances and resistances.
Calculations can be made much simpler by knowing the short-circuit power Ssc at the point that joins the distribution system. Knowing Ssc at this point, the equivalent Za impedance upstream from this point can be calculated using the formula:
There may not be a single source of voltage, but rather several sources in parallel, in particular, synchronous and asynchronous motors, reacting like generators upon the occurrence of short circuits. Three-phase short circuit current is generally the strongest current that can flow in the system.
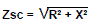
Two-phase short circuit current is always weaker (by a ratio of e/2, i.e. approximately 87%).

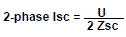
3.Phase-to-earth short circuit current (single-phase)

The value of this current depends on Zn impedance between the neutral and earth.
This impedance can be virtually nil if the neutral is directly grounded (in series with the earthing connection resistance) or, on the contrary, almost infinite if the neutral is ungrounded (in parallel with the system’s phase to earth capacitance).
Calculation of this unbalanced short-circuit current requires the use of the symmetrical components method. This method replaces the real system by superimposing 3 systems: positive Z1, negative Z2, zero sequence Z0.
The value of the phase-to-earth fault current Io is:

This calculation is required for systems in which the neutral is earthed by a Zn impedance. It is used to determine the setting of the “earth fault” protection devices which are to intervene to break the earth fault current.
In practice :