Abstract
Content
- Introduction
- 1. Theme urgency
- 2. Goal and tasks of the research
- 3. Take-off methods
- Conclusion
- References
Introduction
Magnetic phenomena were known in the ancient world: the compass was invented more than 4000 years ago, and to the XII century it became known in Europe. However, only in the XIX century was discovered a connection between electricity and magnetism, and the idea of the magnetic field. The first experiments showed that between electric and magnetic phenomena there is a connection, there were experiments of a Danish physicist H. Oersted (1777-1851). In his famous experience described now in all the textbooks of physics and held in 1820, he found that the wire along which the current acts on a magnetic needle.
Oersted not only had their experience, but also reached the correct conclusion, that the electric conflict is not restricted to the conducting wire, and has a pretty extensive sphere of activity around the wire. This can be understood as the effect of the current is not only inside the wire but around it.
1. Theme urgency
The human body is affected by external environment: changes in pressure, moon phases, eclipses, radiation, magnetic fields which create the transformers. Every day in the steel industry used all sorts of stoves, which emit fields affect the human body. For safe operation it is necessary to know the threat posed by plant, which serves the staff and to protect them from the negative impact on their body.
2. Goal and tasks of the research
The aim of the study is the field generated by an industrial vacuum furnace, SNWA.
- Remove the actual parameters from the vacuum unit.
- To build a model according to the obtained results in the software.
- To compare the results of a typical installation and screened.
3. Take-off methods
A stationary electric charge and a stationary magnetic pole do not interact with each other. Between them there are no forces of attraction or forces of repulsion, these forces do not arise between them under any circumstances if they remain stationary relative to each other. However, it is sufficient to cause an electric charge or magnetic pole is in motion, as at once appears between them interaction force tending to rotate them relative to each other. This force increases with increases in the speed of their relative movement and, in addition, depends on the angle formed by the directions of their movement. When moving charges and moving charges associated with the electric field, a magnetic field. Around moving electric charge appear closed lines of magnetic forces; these lines are in the form of concentric circles cover the trajectory of the charge. When the conductor passes current around the conductor formed a closed line of magnetic induction, concentric rings covering the conductor: the magnetic forces at all points of the plane perpendicular to the axis of the direct current, directed along the tangent to the circle drawn from the point of intersection of the axis of the current plane, and lie in this plane. The forces acting in the magnetic field of a current, decrease with increasing distance from the axis of the current. The direction of the magnetic field associated with the direction of the current rule of thumb: If forward movement of the screw means in the direction of the current in the wire, the direction of rotation of the handle the thumb will correspond to the direction of the magnetic field lines. When the forward movement of the thumb in the direction of the current rotational movement of the arm thumb indicates the direction of the magnetic field lines of a current. Rule of thumb can be used to determine the direction of the current in the direction of its magnetic field lines. If you screw the corkscrew in the direction of the magnetic line, then the direction of rotation of the handle will indicate the direction of current in the circuit, covering the power line. Along with the corkscrew rule often use the following rule: if you look at the direction of the current, the magnetic lines are directed in the direction of movement of the clock hands. The form of a magnetic field in space for the case of straight-line current can be represented as a series of coaxial cylindrical surfaces having as their axis the axis of the current. The magnetic forces in the field of direct current in any point is always directed along the tangent to the cylindrical surface passing through this point and perpendicular to the generatrix, which is the point.
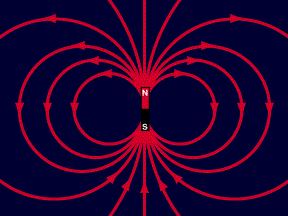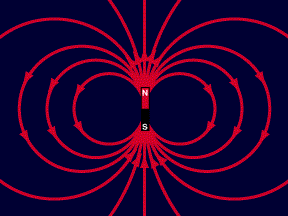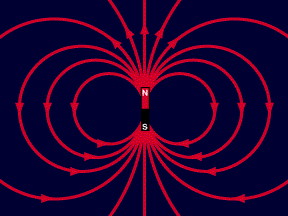
Figure 1 – magnetic field.
Consider the field of the permanent currents in the dielectric medium. Both in the medium surrounding the conductors with a constant current, and the conductors inside there are magnetic and electric fields. These fields are stationary. Outside the source of EMF electric field the direct currents is the same as the electrostatic field, irrotational. This field is potential, i.e. its characteristics can be a function of coordinates. Thus, the electric field in the dielectric medium surrounding the conductors with constant currents. So, in a dielectric medium, this eld does not differ from electrostatic, but the boundary conditions at the surface of conductors do not correspond to those in electrostatics. In the electrostatic problem the surface of each conductor is a surface of equal potential. During the flow of DC current in the conductor there is an potential drop, and hence the surface of the conductor will not be equal to potential. Since the surface of the conductor appears tangential component of field intensity in the direction of the lines of current, lines of the electric field in the dielectric medium come to the surface of the conductor is not at a right angle. This fact considerably complicates the calculation of the field, however, in almost all cases it can not be ignored, because usually the voltage drop along the conductors at a length comparable to the distance between conductors is negligibly small compared with the potential difference of the conductors. when considering the field around the wires can be neglected without fear of this make any noticeable error. In this case, the boundary conditions at the surface of conductors are identical to the conditions in electrostatics. Therefore, in consideration of the electric field in the dielectric medium surrounding the conductors with constant currents, you can use the solutions obtained in the consideration of electrostatic problems.
Let us now consider electromagnetic processes accompanying atmospheric discharge, for example lightning strike to a lightning rod. However, as a rule, it is necessary to consider the heterogeneity of the electric properties of the medium, as well as their change. In mathematical modeling of the investigated processes can also change the geometric configuration of the AI of the electrical parameters of the discharge structure. Note that as the height of the lightning rod, and the length of the leader channel of the lightning is several orders of magnitude greater than their diameter. Therefore, when using the finite difference method, the spatial grid step h is usually chosen much larger than the radius r0 , for example, equal to the average step length during the growth bit structure (lightning). In accordance with the method of finite differences area of interest with thin conductive wire is divided into cells so that the nodes of the computational grid lie on the boundaries of the media and on the axis of the wire. Within each cell, except those which are adjacent to the wire, the properties of the dielectric environment rely homogeneous. The job of the electrical properties of the medium is by assigning values to the relative the dielectric constant ? and the conductivity ? on cells of the design scheme. The boundary conditions depend on the type of field-generating system. Writing equations for each node of the computational grid in a differential form and boundary conditions, we obtain the system of equations, which the author proposes to solve by iteration method. To account for the presence in the analysis area of a thin wire of length, we introduce a system in which the in particular wire located along the Y-axis perpendicular to the ground and one end touches the surface. The coefficients of the tensor components of the nodes located on Let us consider the system of corona wires above the grounded surface. For the calculation of electric fields and charges encountered in this system we use the system of Maxwell's equations describing the electric field distribution and charge: Boundary conditions: a) on the surface of the wires is set to a constant potential; b) on earth surface potential and DC equal to zero; b) in the case of the crown, on the wire always set to the same value the normal component of the tension the electric field for which the known empirical formula Peak. In accordance with these assumptions, the system splits into two independent systems of equations. Equations initial approximation: where is the unperturbed potential. The equations define a field in the absence of the crown. To put the usual boundary conditions for potential on the ground and the wires. Reversible in this context, attention to one feature — the lack of boundary conditions for ? that does not cause difficulties in special cases when analytical solution is possible, but numerical solution of this circumstance significantly complicates the calculation algorithm. In these difficulties it is proposed to overcome the introduction of a new function ?: And on the surface of the earth. Solving the equation from the earth's surface, we get the value?a on the surface of the wire. The charge density along the field lines: Knowing the charge density, it is possible to find a function ? along the lines of force, solving the equation of the wire surface. Thus, on the basis of the mathematical models, the study of electric fields can be carried out on the basis of the solution of external boundary value problem for the Laplace equation with boundary conditions of Dirichlet and Neumann.
Conclusion
The article considers the complex problems associated with mathematical modeling of electric fields. Developed the inversion method used in the solution of external boundary value problems with boundary conditions of Dirichlet and Neumann problems in two-dimensional and three-dimensional half-space. It justifies the use of values at the points of both parts of a two-part region in the analysis of the results of the decision. Developed computational algorithms and based on them the software for modeling the electric fields based on the method of inversion. Made test calculations showed that the developed algorithms reduce the required computing resources. Discovered a significant increase in the accuracy of the test calculations according to the developed inversion method compared to approaches that use basic characteristic boundary conditions. In addition, computer modeling of electric fields with the application of the developed inversion method to fit the results computational and physical experiments conducted by other authors, both qualitatively and quantitatively. In contrast to the previously performed work of similar subjects in this study are solved by an external boundary value problem in two-dimensional and three-dimensional half-space with the task of boundary conditions on closed and semi-infinite and infinite surfaces in accordance with the method of inversion, taking into account the values in both parts of the two-phase region and increases the speed of solving such class of problems when modeling the electric fields.
References
- Калашников С.Г. Электричество: Учебное пособие. –М.: Наука. 1985. –576с.
- Теоретические основы электротехники : В 3-х т. Том 3. – 4-е изд. / К. С. Демирчян, Л. Р. Нейман, В. Коровкин, В. Л. Чечурин. – СПб. : Питер, 2004 г. – 377 с.
- Потапенко, А. Н. Математическая модель и численный метод исследования электрических полей высоковольтных воздушных линий электропередач / А. Н. Потапенко, Е. А. Канунникова, О. В. Донева // Известия высших учебных заведений. Северо-Кавказский регион. Технические науки. – 2008. – № 5. – С. 41-45.
- Попков, В. И. Коронный разряд и линии сверхвысокого напряжения : избранные труды / В. И. Попков. – М. : Наука, 1990. – 256 с.
- Рязанов, Г. А. Опыты и моделирование при изучении электромагнитного поля / Г. А. Рязанов. – М. : Наука, 1966. – 191 с.
- Канунникова, Е. А. Метод инверсии внешней бесконечной области применительно к задачам электростатики / Е. А. Канунникова // Образование, наука, производство и управление : сб. докл. междунар. науч.-практич. конф. 22–23 ноября 2007 г. – Ст. Оскол : Изд-во СТИ, 2007. – С. 101-104.
- Свешников, А. Г. Лекции по математической физике /А. Г. Свешников А. Н. Боголюбов, В. В. Кравцов. – М.: Изд-во МГУ, 1993. – 352 с.
- Колечицкий, Е. С. Расчет электрических полей устройств высокого напряжения / Е. С. Колечицкий. – М. : Энергоатомиздат, 1983. – 168 с.
- Yang, Y. The strip simulation method for computing electric field on conductor surfaces / Y. Yang, D. Dallaire, J. Ma, F. P. Dawalibi // Power and Energy Systems (EuroPES 2003) : proceedings of the Third IASTED International Conference 3-5 sept. 2003, Marbella. – 2003. – P. 353-357.
- Справочная книга радиолюбителя–конструктора/ А.А. Бокуняев, Н.М. Борисов, Р.Г. Варламов и др. Под ред. Н.И. Чистякова. – М.: Радио и связь, 1990. - 624 с.
