Abstract
Contents
- Introduction
- 1. Theme urgency
- 2. Goal and tasks of the research
- 3. Asynchronous mode in the power grid
- 3.1 General Asynchronous Information
- 3.2 Established asynchronous mode
- Conclusion
- References
Introduction
The complication of the structure of electrical systems, due to their development, has a decisive effect on the regimes of these systems and leads to a significant increase in the amount of research and calculations associated with identifying the operating conditions of power systems when the normal mode is disturbed. Ensuring the dynamic stability of synchronous generators in emergency modes is one of the most pressing problems in the management of modern power plants and systems [1].
The study of processes in the electric power system in case of violation of dynamic stability is in itself a rather laborious task, the solution of which requires a significant expenditure of resources and time. This is due to the fact that the flow of an asynchronous mode is influenced by many different factors, such as: network configuration, type of regulation used, current operation mode, and so on.
1. Theme urgency
In case of large disturbances in electrical power systems, a violation of dynamic stability may occur. Real conditions of operation of power systems, violation of dynamic stability leads to the appearance of a two-frequency and multi-frequency asynchronous operation.
Asynchronous mode is one of the most severe emergency modes, its occurrence may trigger the subsequent development of cascade accidents, lead to irreversible damage to generators and turbines, and cause irreparable damage to other electrical equipment. In addition, the asynchronous mode of one machine can lead to a breakdown of the other generators of the integrated power system [2–4].
In this regard, the relevance of the problem of assessing the admissibility of asynchronous modes increases.
Together with this task it becomes necessary to simulate dynamic processes occurring in the network for their further research. To improve the accuracy of the analysis of the admissibility of AR, it is necessary to use mathematical models that most correctly describe the behavior of the electric power system in a transient mode.
2. Goal and tasks of the research
The aim of the work is to analyze and study the approaches and methods for assessing the admissibility of asynchronous modes in electrical systems.
The main objectives of the study:
- The study of existing methods of analysis of the admissibility of the asynchronous mode, the assessment of their advantages and disadvantages.
- Investigation of asynchronous modes.
- Investigation of mathematical models of the generator for modeling the asynchronous mode.
Research object: the analysis of the admissibility of synchronous mode.
Research subject: a model of a section of an electric power network, implemented in the PowerFactory software package.
As part of the master's work, it is planned to obtain relevant scientific results in the following areas:
- Identification of the optimal mathematical model of the generator for the analysis of the admissibility of the asynchronous mode.
- Identify the criteria for the admissibility of asynchronous mode.
- Modification of the known methods of analysis of the asynchronous mode destabilization.
3. Asynchronous mode in the power grid
3.1 General Asynchronous Information
Asynchronous mode – the mode of the generator, in which the speed of rotation of the rotor deviates significantly from the synchronous speed of the network. This mode is characterized by the following changes:
- deep and stable fluctuations of mode parameters (voltages, currents, power);
- a change in the EMF mutual angle;
- occurrence of frequency difference between parts of the power system.
Asynchronous mode can cause damage. equipment at the power plant, a massive power outage of consumers, a complete loss of control of the power system mode and the collapse of the power system.
The power plant equipment operates in the mode of periodic changes of currents and voltages, while eddy currents occur in the closed rotor circuits, causing it to overheat.
Asynchronous mode may occur in the following cases:
- when overloading power lines under the terms of static stability;
- in case of violation of dynamic stability;
- non-synchronous switching on of power lines and generators;
- at loss by the excitation generator.
3.2 Established asynchronous mode
Asynchronous mode is mainly characterized by the difference in the speeds of rotation of the EMF vector of the generator and the system. The difference of these frequencies is determined by the slip.
The steady-state asynchronous mode occurs when a balance occurs between the turbine moment and the asynchronous moment, characterized by the slip S, which is determined by the intersection point of the characteristics of the turbine moment and the asynchronous moment (Fig. 1).
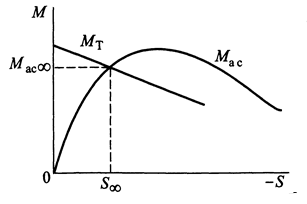
Figure 1 – Determination of slip in steady state
If the machine dropped out of synchronism remains excited, a synchronous torque will also act on the generator shaft. This alternating moment will cause a periodic change in the speed of rotation of the rotor in asynchronous mode and as a result of slip pulsation (Fig. 2).
At the same time, the mode parameters change significantly.
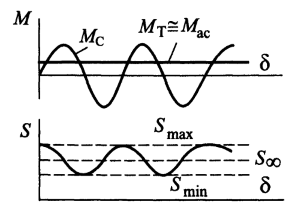
Figure 2 – Change of moments and slip in asynchronous mode
Conclusion
At the time of writing this essay the master's work is not yet completed. Estimated completion date: May 2019. Changes will be made to this abstract as work progresses. The final work will be available from the author or his manager after the specified completion date of writing.
A detailed consideration of the general provisions on the admissibility of asynchronous modes is planned. Analysis of optimal mathematical models of a synchronous generator.
As part of the work is planned to perform:
- simulation of asynchronous modes caused by various disturbances in the EPS in the PowerFactory software package;
- analyze the validity of asynchronous modes;
- analyze the influence of various factors on the flow of the asynchronous mode.
References
- Андерсон П. Управление энергосистемами и устойчивость / Андерсон П., Фуад А.; пер. с англ. под ред. Я. Н. Лугинского. – М.: Энергия, 1980. – 568 с.
- Савалов С. А. Противоаварийное управление в энергосистемах // Савалов С. А., Семенов В. А. М.: Энергоиздат, 1988, 416 с.
- Pourbeik P. The anatomy of a power grid blackout // Pourbeik P., Kundur P. S., Taylor C. W. IEEE Power and Magazine, 2006, Vol.4, No.5, p.22-29.
- Makarow Yu. V. Blackout prevention in the United States, Europe and Russia // Makarow Yu. V., Reshetov V. I., Voropai N. I. Proccedings of the IEEE, 2005, Vol.93, No.11, p.1942-1955.
- Федотов А. И., Лопухов В. М., Соколов В. А., Зайцев Д. А. Автоматика ликвидации асинхронного режима на блоках с турбогенераторами средней мощности / Известия высших учебных заведений. Проблемы энергетики. 2007. № 7-8. С. 66-71.
- Веников В. А. Переходные электромеханические процессы в электрических системах: Учеб. для электроэиергст. спец. Вузов. – 4-е изд., перераб. и доп. – М.: Высш. шк., 1985. – 536 с, пл.
- Наровлянский В. Г., Ваганов А. Б., Иванов И. А. Результаты испытаний устройства ликвидации асинхронного режима АЛАР-М / Релейная защита и автоматизация. 2010. № 1. С. 16-18.
- Камель Н. А. Разработка и исследование методов анализа асинхронных режимов в энергосистеме и протавоаварийной автоматики для их ликвидации: дис. дис. к. т. н: 5.14.02: защищена 1.02.1995 – М., 1995.
- Матвеев И. В., Пастухов B. C., Иванов К. М. Автоматика ликвидации асинхронного режима (АЛАР) / Вологдинские чтения. 2008. № 69. С. 96-98.
- Ульянов С. А. Электромагнитные переходные процессы в электрических системах / Ульянов С. А. – 2012. – 512 с.
- DIgSILENT Technical Documentation Synchronous Generator. Published by DIgSILENT GmbH, Germany. Copyright 2007. All rights reserved. Unauthorized copying or publishing of this or any part of this document is prohibited. TechRef ElmSym V1 Build 331 30.03.2007
- Сивокобыленко В. Ф. определение параметров схем замещения синхронных машин / В. Ф. Сивокобыленко, В. К. Лебедев // Научные труды ДонГТУ, серия Электромеханика и электроэнергетика, вып 1. – Донецк – 1996. – С. 98-102.
