Contents |
| 1. Urgency of carried out research |
| 2. The basic concepts of the signature analysis |
| 3. Multiplechannel signature analyzer |
| 4. The conclusion |
Urgency of carried out research |
Wide distribution of radio-electronic devices with application digital processings of signals are caused with heightened interest to questions diagnosings of their technical condition. One of versions diagnosings of digital units and blocks is test diagnosing, which application at a design stage and manufacturing of digital units allows to define their correctness functioning and to carry out procedure of search of malfunctions. At to development of test diagnostics there is a complexity in definition reference reactions at testing existing schemes, in definition optimum number of control points for removal of target reaction the diagnosed digital scheme. It can be made or creating the prototype the developed digital device and spending its diagnostics hardware methods, or carrying out modelling on the COMPUTER as the digital device, and process of diagnostics. The most rational The second approach which assumes creation automated is systems of diagnostics [5].
The basic concepts of the signature analysis |
In present time in new technics of testing of digital schemes signature the analysis is applied most often. It has been predetermined by several the reasons [5], for example such: uniformity of the law of distribution probabilities Pi Not detection of a mistake of frequency rate i and set nndetectable mistakes Vi Frequency rates i includes improbable configurations of erroneous bats in sequence of data.
Main idea of the signature analysis at use of a method of division of a polynom on the polynom is based on performance of operation of division of multinominals. In quality of a dividend the dataflow, formed on an output is used the analyzed digital unit which can be presented as multinominal p (x) degrees L-1, where L - length of a stream. As a divider serves primitive not resulted{brought} polynom p (x), as a result of division on which it turns out private q (x) and rest S (x), connected by a parity
| p(x)= q(x) p(x)+ s(x), |
Where the rest s (x), a representing polynom of a degree, smaller than m=degP (x), refers to as the signature [9]. We shall result the scheme the signature analysis on an example of a polynom x5+x4+x2+1
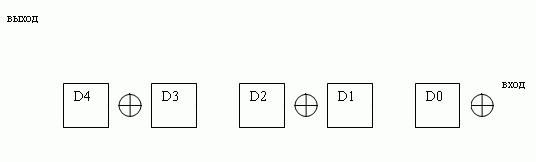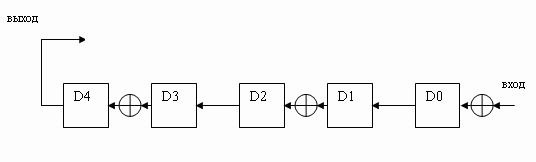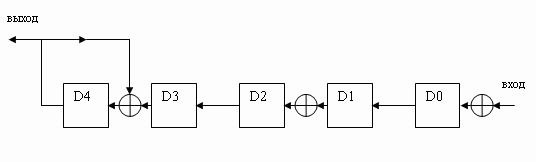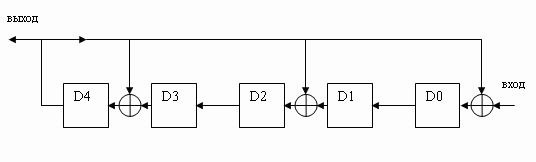 |
| Figure 1 - Signature analyzer constructed on a method of division of polynoms. |

|
| Figure 2 - Signature analyzer constructed on a method of convolution. |
Multichannel signature analyzer |
Let's assume, that the single-channel analyzer is used for the analysis of the digital unit, having channels, and target sequences in this case will be transformed to one sequence of a kind
 |
| where |
 |
- value of a binary symbol on-th output of digital unit in-th step of its work, and clock frequency of work of the analyzer in time above frequency of synchronization of the investigated unit. Thus in each step of work of the analyzer on its input consistently, since the first output, values act |
 |
Functioning of the single-channel analyzer in a multichannel mode when the quantity of channels is equaled, described by system of the equations |
 |
Where numerical value of factors is defined on the basis of following system of the equations |
 |
Factors |
 |
Will be defined as follows: |
 |
The conclusion |
The core
property of the widespread algorithms of formation test
sequences is that as a result of their application
sequences of very big length generating. Therefore on outputs
the checked digital scheme its reactions having the same length are formed.
naturally there are problems of their storing and storage. The elementary
the decision allowing considerably to reduce volume to the information about
reference target reactions, reception of integrated estimations is,
having smaller dimension.
That
to apply a method of compact compression of testing, it is necessary
it is rational to choose algorithm of formation of test sequences and
method of compression of the information.
| to beginning |