Abstract
 Let the cargo is moved so that it accelerates at first, moving down, then time moves at a constant speed, then slows and finally stops. At the end of time, pausing, he begins to move in the opposite direction, passing the same stage of the movement cycle.
This chart illustrates in Fig. 1, which illustrates the change in velocity  and acceleration  of the load during one cycle of operation of descent-recovery. This spurt in the change of accelerations taken so large that at the points 0, 1, 2, 3 there are abrupt «fractures» the velocity  function and the acceleration  of change «step».
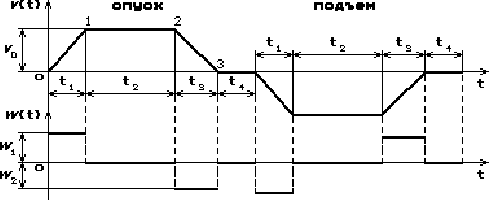
Figure 1 - Graphs of velocity and acceleration without jerk limitation
Figure 2 - Diagrams of velocity and acceleration with jerk limitation
Fig. 2 illustrates the change in variable speed and acceleration of the load with a small push to ensure a smooth change of speed at the points 0,1,2,3 (ie at intervals  ,  ,  and  ) Trapezoidal and nature of changes in acceleration . The function changes breakthrough here is not shown, but we can say that it represents a step line and it differs from zero only at intervals , , and .
Effect of magnitude leap in the dynamic state of the crane shown in Figure 3. Curve 1 is for the option, when all  is so great that the chart speed (at the top of the figure) consists of one broken line. Curve 2 is constructed for the case when the value calculated by the formula «optimal jerk»
and at the same time, as can be seen, there is no oscillatory nature of the function of displacement. The very same function  as it copies the acceleration  of figure that confirms the above.
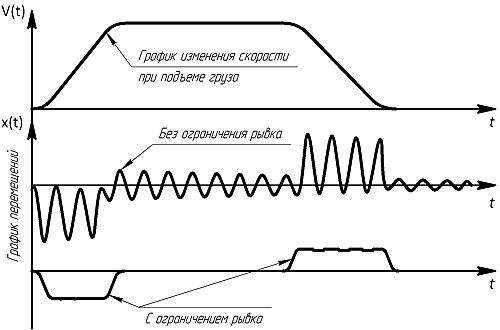
Figure 3 - Dynamic processes in the descent of the load at different breakthrough
The Master's work is not completed yet. Final completion: December 2009. Full text of the work and materials on the topic can be obtained from the author or his supervisor after this date.
References
1. Лобов Николай Александрович / Динамика грузоподъемных кранов, М.: Машиностроение, 1987. - 156 с.
2. Гелетий Владимир Николаевич / Разработка динамических моделей и совершенствование расчета башенных кранов. Автореф. дис., (01.02.06). – Львов, 1985. – 18 с.
3. Дворников Владимир Иванович / Конспект лекций по курсу «Динамика строительных машин», Макеевка 2001. - 63 с.
4. Улучшение эксплуатационных характеристик грузоподъемных кранов / Сарадачев, Владимир Иванович, Сердюк, Э.И., Железняк, В.А. – М.: Недра, 1992. – 238 с.
5. Александров М.П. / Подъемно-транспортные машины – М.: Высшая школа, 1985. - 514 с.
6. Ваинсон А.А. / Подъемно-транспортные машины. Машиностроение, 1975. - 431 с.
7. Иванченко Ф.К. / Конструкция и расчет подъемно-транспортных машин. – Киев: Вища школа, 1983. - 351 с.
8. Кузьмин А.В., Марон Ф.Л. / Справочник по расчетам механизмов подъемно-транспортных машин. – Минск: Высшая школа, 1983. - 351 с.
|
